On a quiz there are four multiple-choice questions worth 3 points each and two true/false questions worth 1 point each. Each multiple-choice question has five possible choices. If a student randomly guesses on each question, what is the expected value of the student's score on the test? 1.8 2.8 3.4 5.4 * =

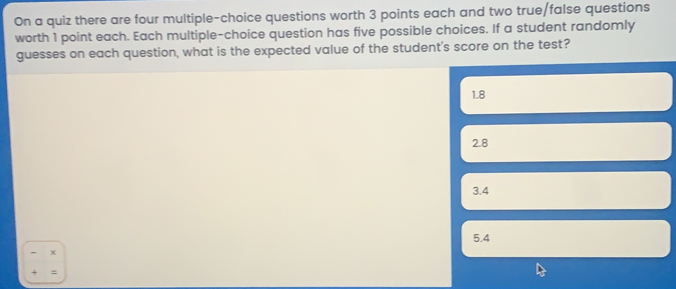
Answer: Expected value would be [tex]\dfrac{17}{5}[/tex]Step-by-step explanation:Since we have given that Number of multiple choice question = 4Number of true false questions = 2Number of points for each multiple choice question = 3Number of points for each true false question = 1Number of choices in multiple choice = 5Probability of getting correct answer in multiple choice question = [tex]\dfrac{1}{5}[/tex]Probability of getting correct answer in true false question = [tex]\dfrac{1}{2}[/tex]Expected value of the student's score on the test would be [tex]E(x)=\sum xp(x)\\\\E(x)=4\times 3\times \dfrac{1}{5}+2\times 1\times \dfrac{1}{2}\\\\E(x)=\dfrac{12}{5}+1\\\\E(x)=\dfrac{12+5}{5}=\dfrac{17}{5}[/tex]Hence, expected value would be [tex]\dfrac{17}{5}[/tex]